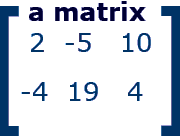The Teenage Brain

Muliplying Matrices

Here Jordan Bayne explains the concept of dimension statement. I can really see he knows what is going on! Really nice!!!

Muliplying Matrices: "Before you can begin to multiply matrices you need determine whether or not the matrices can be multiplied. To do that you must write a dimension statement.

[3 4] [1 2 3]
[5 6] [4 5 6]
[7 8]
The dimension statement for this would be 3X2 times 2X3.

• The numbers on the inside (in red) must be the same to carryout the multiplication process
• The numbers on the outside (in blue) tell what the dimension of the solution will be. In this particular problem, the solution will be a 3 X 3 matrix.

If the two matrices can be multiplied, you then multiply row X column and add the sum of the products to get the solution to the problem.

"

Int Algebra II - Erin Johnson: Can you multiply Matrices?

Good example of what I am looking for...click on the link!

Int Algebra II - Erin Johnson: Can you multiply Matrices?: "To determine whether or not matrices can be multiplied you first have to write a dimensions statement. Example of a dimensions statement: [..."

INT ALG II - Alex Nguyen: Multiplying Matrices

To see what muliplying matrices are about...


INT ALG II - Alex Nguyen: Multiplying Matrices: "Scalar matrix multiplications Distribute the outside number for all the numbers on the inside. When multiplying matrices. We must note..."

Dimensions of a Matrix

Here is a good one from Alex Reid!!!

Dimensions of a Matrix: "

The dimensions of a matrix refer to the number of rows and number columns of a given matrix.

The rows of a matrix are the numbers going horizontally.

The columns of a matrix are the numbers going vertically.

To find the dimensions of a matrix you must multiply the (number of rows x the number of columns). The dimensions for the matrix above would be (2 rows x 3 columns). The dimension of the matrix is 2 by 3 or (2x3).

Dimensions of a Matrix

This looks like a good start!  However, this student needs to add something about the square matrices and the matrix that is the identity matrix.  These are important concepts that you need to be able to identify!

Dimensions of a Matrix: "To count matrices you count row X column

This matrix is one row by three columns, so it is a 1 x 3 matrix.

This matrix is three rows by three columns, so this is a 3 x 3 matrix.

This matrix is three rows by two columns, so it is a 3 x 2 matrix.

This is another three rows by three columns, so this is a 3 x 3 matrix.

"

Math is not linear on Prezi

Matrice multiplication

To multiply matrices you have to do ROW X COLUMN sum of the products.....

You will repeat this process several times...

Matrix Operations

This is the information used to introduce matrices

We started matrices!

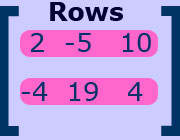
You will need these images to describe how to find the dimensions of a matrix.

Row X Column, identify the ones that are square and the identity matrix!  Find the video on brightstorm, look on purplemath or any other math website to find other examples...use google images to see if you can find standing, price lists, anything that could be represented using matrices!!!

This picture is for fun....

These images you need for blogging!

Count the rows and columns, show how to identify the dimensions

Shrek and you are testing tomorrow!

Good Luck!  On your Chapter 1 test....chat with Coach Hill by finding rthill3 on Google Talk

Types of Systems: GREAT!!!

Types of Systems:

Case 1	Case 2	Case 3

Independent system: one solution point	Case 2	Case 3

Independent system: one solution and one intersection point	Inconsistent system: no solution and no intersection point	Case 3

Independent and consistent system: one solution and one intersection point	Inconsistent system: no solution and no intersection point	Dependent system: the solution is the whole line

"

Error Analysis - This one is perfect!

• For this particular problem the value of x is going up by 5, so the slope should be 10/5 or 2 and not 10/1. By inserting the points, the final equation should be solved. With this answer, y is not equal to 9+10x in the t-chart.

• In order for a particular point to be the solution to a system, it has to solve both equations. So in this case (1,-2) solves the first equation, but not the second equation of the system.

• For problem #22 the shading is correct, but the line should be a dotted line, not a soild line. For problem #23 the solid line is correct, but the shading should be above the line, not below it.

• For problem #20 the shading is correct, but the line should be a dotted line and not a solid line. For problem #21 the sloid line is correct, but the shading should be below the line, not above it.

"

Study Skills

Big week...here are all the assignments in Chapter 1

1)  Quiz on writing equations

2)  Quiz on systems

3)  Quizstar - 3 Quizzes

4)  Blogging - 3 Blogs

5)  Chapter 1 Test

     Extra credit packets - Misc. worksheets from the Chapter

This is how to work error analysis problems

The x's are going up by 5...not by 1. Therefore the slope would be 10/5...which equals 2 not 10/1.  

Plus the final equation should be solved by plugging in the points, y is not equal to 9 + 10 * x in the t-chart!

Systems of Equations with 3 Variables

Needed for next week!

Error Analysis Blog

Use these Pictures to Blog on Error Analysis

1) 

2) 

3) 

4)

Emailing to my blogs

Think I have figured out how to just email my blogs in...you can try it too!  Goto to the Designs tab and the Settings, choose email or phone to update!

--

Robert Hill

 

www.rthill3.com

(678)640-3180 Cell

(678)615-6274 Home

(770)564-1438 Work

Beautiful Picture of my daughter and friends!!!

Don't know if you heard them, but they were having a great time today!

Perpendicular Lines

This is an example from my Integrated Algebra I class....notice how I made corrections on their blog!  Notice how they have a number for each line of the picture

1. Angle one is equal to angle 

2. The lines are parallel

3. Angles 1 and 2 are set equal to 90

4. The lines angles are supplementary

"

Systems of Equations

Systems of Equations: "Consistent independent equations have one solution (x, y), which is where the two lines intersect. Consistent dependent equations have infinitely many solutions because they are the same line and inconsistent equations have no solution because they are parellel.

I like this one...a good example of the different types of systems!

y=a|x-h|+k

 y=a|x-h|+k
the vertex on your graph will be (H,K)

the A tells you whether the V will open up or down,so if a is negative is will open down,and if it's positive, it will open up

Your A also tells you the slope,so if your a is 1 your slope would be 1/1 So up 1 over 1, up 1 over 1 etc.

the H moves the V right or left (opposite,so if you H is negative it would move right,and if it was positive it would move left)

the K moves the V up or down

The green graph is the graph of the function we want. It is a translation of the blue graph moved one unit down and 2 units left

Here is a good example of what I am looking for...nice job!!!